IM Certified® Blog
Using Math Routines to Build Number Sense in First Grade
By Allison Van Voy When I started teaching four years ago, I had no idea how important number sense was to a student’s math understanding. I was fresh out of college, brand new to teaching, and number sense was not a...
Sometimes the Real World Is Overrated: The Joy of Silly Applications
By Charles Larrieu Casias One of the cool things about math is that it can provide powerful new ways of seeing the world. Just for fun, I want you to open up this lesson from the grade 8 student text. Take a quick skim....
Instructional Materials Matter: Interpreting Remainders in Division
By Jody Guarino We know instructional materials play a key role in student learning experiences but how do we ensure our students are learning from coherent high-quality instructional materials that engage them in critical...
Adapting Curriculum For Students to Know, Use and Enjoy Fractions
By Melissa Greenwald You know it is time for a change when half of the students in class are lost by the third lesson of a new unit. I teach third grade in a charter school in Philadelphia. We use Go Math! and each year I...
Learning Goals and Learning Targets
By Jennifer Wilson One of your students is asked, “What are you learning about today in class?” How does your student respond? “Nothing” “Math” “The questions on this worksheet” “Deciding if two figures are congruent”...
Warm-up Routines With a Purpose
By Kristin Gray As a teacher, curiosity around students’ mathematical thinking was the driving force behind the teaching and learning in my classroom. To better understand what they were thinking, I needed to not only have...
Adapting Problems to Elicit Student Thinking
By Jody Guarino As a teacher, I constantly wonder how I can elicit student thinking in order to gain insight into the current thinking of my students and leverage their thoughts and ideas to build mathematical...
A Fraction Unit Does Not Always Begin With Lesson 1
By Jared Gilman As I sat down at my local coffee shop to plan my upcoming 5th grade unit on fractions, a wave of dread spread across my body. I started having flashbacks to last winter, when my students’ frustrations with...
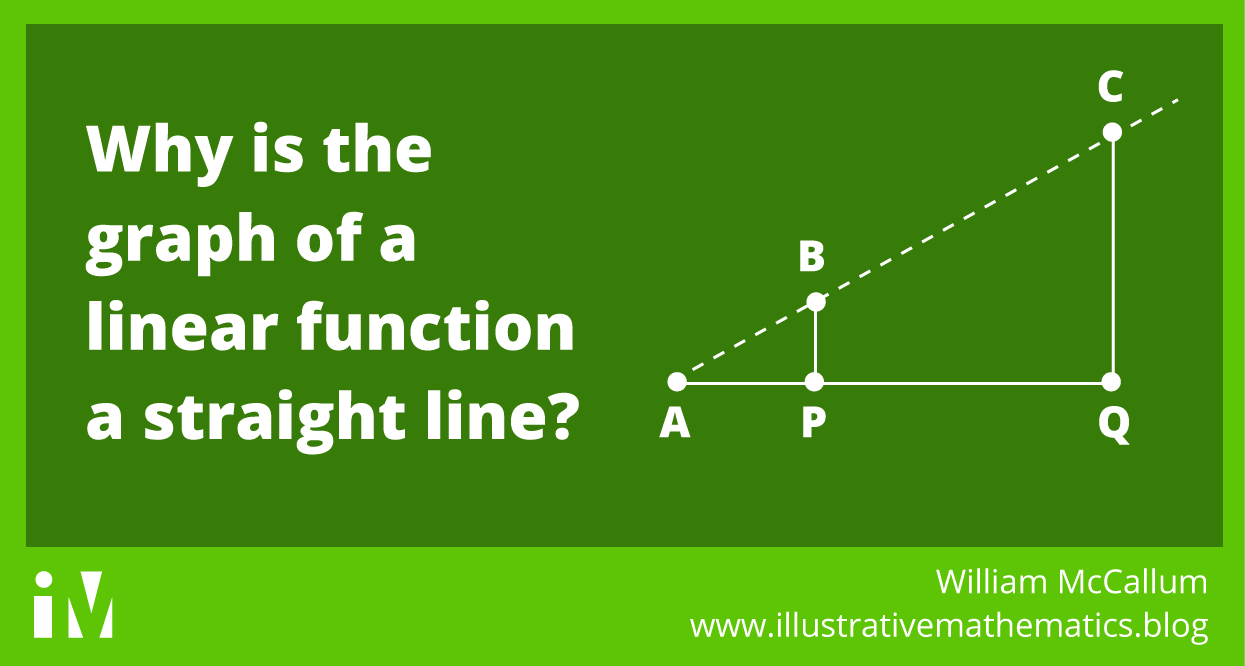
Why is the graph of a linear function a straight line?
By William McCallum In my last post I wrote about the following standard, and mentioned that I could write a whole blog post about the first comma. 8.F.A.3. Interpret the equation $y = mx + b$ as defining a linear function,...
Why We Don’t Cross Multiply
By Kate Nowak (co-authored with Kristin Gray) “Ultimately, the goal of this unit is to prepare students to make sense of situations involving equivalent ratios and solve problems flexibly and strategically, rather than to...
The Illustrative Mathematics Team Reflect on the 5 Practices
The entire Illustrative Mathematics team spends a lot of time reading about teaching and learning. Most recently, we have been reading—some of us rereading—and reflecting on the 5 Practices for Orchestrating Productive...
Using the 5 Practices with Instructional Routines
By Robin Moore As a coach, how can I help teachers structure their lesson-planning in order for students to unpack their mathematical understandings? This question is always at the forefront of my mind as I reflect on my...
Vocabulary Decisions
By Bowen Kerins A wide-ranging team worked together to develop the Illustrative Mathematics Grades 6–8 Math curriculum. Many of the authors were and are experienced teachers of Grades 6–8, while others are experienced high...
How the 5 Practices Changed my Instruction
By Alicia Farmer I am the type of teacher you want on your teaching team. I am the person that can remember vast amounts of details, predict potential obstacles, and meet any and all deadlines. My organized personality is...
The 5 Practices Framework: Explicit Planning vs Explicit Teaching
“Whether we’re asking students to analyze a historical event, reflect on a text, or work toward a scientific discovery, we need to give students a chance to dig into the ideas on their own first.” By Kristin Gray I’ve come...
Not all contexts have the same purpose
By Nik Doran We sometimes use familiar contexts to understand new mathematical ideas, and sometimes we use familiar mathematical ideas to understand what is going on in a context. We do both of these things by looking for...
Welcome to the new Illustrative Mathematics blog!
In continually moving forward with our vision of creating a world where learners know, use and enjoy mathematics, the Illustrative Mathematics team is so excited to announce the launch of our official blog! Our blog will be...
Info Gap Cards: The Hidden Gem
By Sadie Estrella May 2016 seems so long ago. I actually had to look it up on a calendar because I really thought it was more than 1.41666years ago. That was when I officially started this journey with Illustrative...
Fraction & Decimal Number Lines
By Kristin Gray Recently, our 3rd, 4th, and 5th grade teachers had the opportunity to chat math for 2 hours during a Learning Lab held on a professional development day. It was the first time we had done a vertical lab and...
Respecting the Intellectual Work of the Grade
By Kate Nowak A thing that I think we did really well in Illustrative Mathematics 6–8 Math was attend carefully to really deep, important things that adults that already know math can easily overlook. For example,...
Assessment Principles in Illustrative Mathematics 6-8 Math
By Bowen Kerins A wide-ranging team worked together to develop the Illustrative Mathematics Grades 6-8 Math curriculum. As Assessment Lead, it was my responsibility to write and curate the Shared Understandings...
Reflection & Discussions in Grade 8, Part 1
By Ashli Black Woo, blogging! As I start work on high school curriculum, I thought I would go back and revisit the grade 8 units that I’ve spent the past 18 months working on and share some of my favorite things. This gives...
Fraction division part I: How do you know when it is division?
By William McCallum and Kristin Umland In her book Knowing and Teaching Elementary Mathematics, Liping Ma wrote about this question and how teachers responded to it: Write a story problem for $1 ¾ \div ½$. [Pause here and...
Truth and consequences: talking about solving equations
By William McCallum The language we use when we talk about solving equations can be a bit of a minefield. It seems obvious to talk about an equation such as $3x + 2 = x + 5$ as saying that $3x+2$ is equal to $x + 5$, and...