By Jody Guarino
We know instructional materials play a key role in student learning experiences but how do we ensure our students are learning from coherent high-quality instructional materials that engage them in critical thinking and provide opportunities to “do math?”
Let’s think about this from the lens of a 4th grade standard, 4.OA.A3: Solve multi-step word problems posed with whole numbers and having whole-number answers using the four operations, including problems in which remainders must be interpreted. Represent these problems using equations with a letter standing in for the unknown quantity. Assess the reasonableness of answers using mental computation and estimation strategies including rounding.
Before addressing how instructional materials may approach this standard, I want to first focus on one part of this standard in terms of student thinking, “Solve multi-step word problems posed with whole numbers and having whole-number answers using the four operations, including problems in which remainders must be interpreted.”
How might students take up the idea of interpreting remainders? What is the intent of the standard? The CCSS Progressions Documents can be helpful in understanding the standards and the intent behind them. For example, here is an excerpt from the Operations and Algebraic Thinking progressions document on remainders:
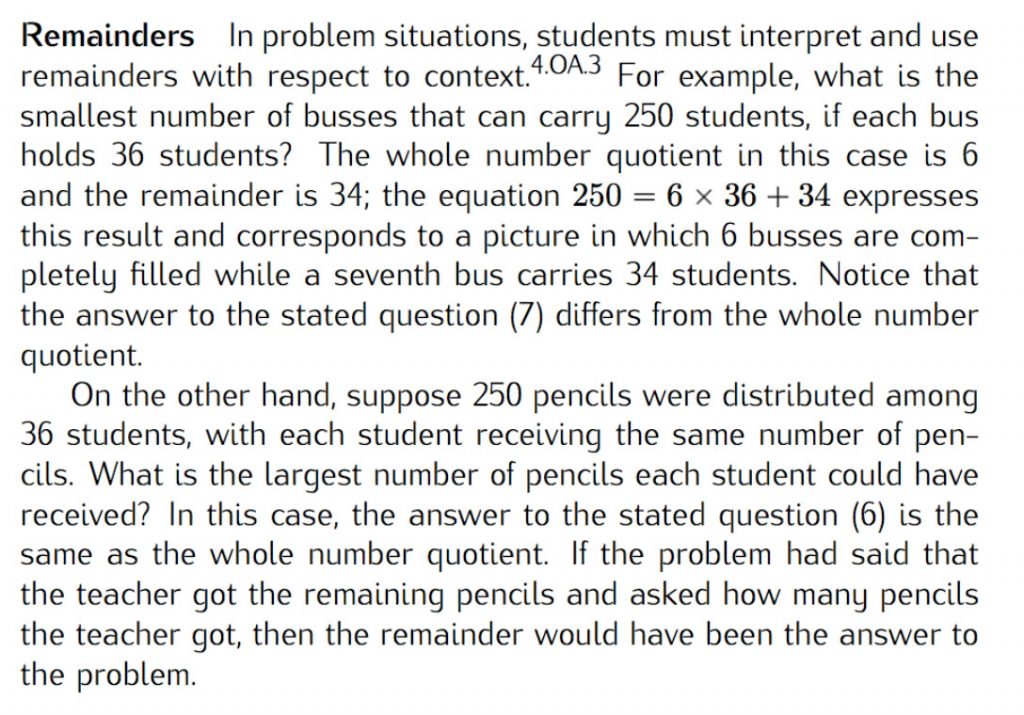
The examples provided are quite distinct. In the first case, students will be carried on buses. With a given number of students (250) needing transport and each bus having a defined capacity (36 students), there will be 6 full busses and a seventh bus that’s partially full. Within this context, students should notice that they need an additional bus (the seventh bus) for all students to be transported. The progression document calls our attention to the fact that the answer to the stated question differs from the whole number quotient. Some may think of this as “rounding up” as the quotient is increased by one so that all students can be transported.
In the second case, a number of pencils (250) are being equally shared by a number of students (36), as evident within the problem statement, “Each student receiving the same number of pencils.” The question being posed, “What is the largest number of pencils each student could have received?” suggests that a remainder doesn’t play a role in this situation, as pencils will be distributed so that students receive the same amount.
These examples caused me to wonder about additional possibilities. For example, what if the first problem was worded, How many full buses will be needed to transport 250 students? In this case, the quotient would be 6 as the question is asking about full buses, not how many buses will be needed to transport all students. The same context could lend itself to the question, How many full buses will be needed to transport 250 students? How many additional students need to be transported? In this case, the quotient would be 6 and there would be 34 remaining students needing to be transported.
Through these examples it is evident that context plays an important role in determining how to interpret remainders. The contexts of students fitting in a bus or pencils being shared lend themselves to whole number solutions. What if the context was different, perhaps children sharing brownies? How do we think about 4 friends sharing 5 brownies so that each friend gets the same amount and all brownies are distributed? Each friend can have 1 brownie and then the fifth brownie has to be taken into account. Since the problem stated, “each friend gets the same amount and all brownies are distributed,” the final brownie needs to be shared. If the brownie is partitioned into four equal parts, one part for each of the four friends, each friend would then get 1 $latex \frac{1}{4}&bg=ffffff$ brownie. In this context, it isn’t unusual to partition a brownie into fractional size pieces whereas it’s problematic to partition pencils (or people) into smaller partitions. Context matters!
Through the lens of the standard and mathematical ideas, what will this look like for students as they build upon their understanding of division and extend that understanding to think about remainders? How are these ideas addressed by instructional materials?
Here are two examples of how instructional materials could address division with remainders: Example 1 from Illustrative Mathematics and Example 2 is a resource someone may find when googling “grade 4 division with remainders.”
Example 1
Task:
- 100 fourth graders are going on a field trip by minibus. Each minibus holds 24 students. How many minibuses will they need to take all of the fourth graders on the field trip?
- 100 fourth graders are going on a field trip by minibus. Each minibus holds 24 people. What is the largest number of minibuses that are completely full?
- Mrs. Slee has 74 inches of ribbon. She can make a bow with 10 inches of ribbon.
- What is the greatest number of bows can she make?
- If she makes as many bows as possible, how much ribbon will she have left?
- Presley has $40. She wants to buy boxes of markers for $6 each.
- How many boxes of markers can she buy?
- How much more money does she need to buy one more box of markers?
- 4 friends want to share 5 brownies so that each person gets the same amount of brownies and all the brownies are used. How many brownies will each person get?
- Identify the division problem that you performed and the remainder in each problem. How did the remainder effect the answer in each problem?
- What did you notice when working with remainders?
Example 2


Pause here to take a minute and think about how these two examples take up these mathematical ideas.
- What might the student experience look like with each?
- As a teacher, what will I learn about student thinking from each?
Example 1 provides students with opportunities for sense-making because math should make sense (MP1). In each problem, students are given a context and based on that context, they determine how to interpret the remainder, aligned with the expectations of the standards and progressions documents. The range of problems provides students with experiences and contexts that include using the remainder in the next step of the problem, partitioning the remainder into fractional parts, rounding the remainder up, and disregarding the remainder. Each element of the task also makes student thinking visible. Students solve problems, interpret remainders, and analyze and reflect on the different ways in which the remainders were interpreted.
Example 2 contains a mnemonic, adding unnecessary, non-mathematical information. DURT takes attention away from the actual mathematics and sense-making. Without context, how do students know how to interpret remainders? Without context, what is the meaning of DURT? Take, for example, the cookie task. The task has promise as four students share 15 cookies, similar to one of the questions in example 1. However, the task doesn’t end with the question.
Why the hint? How does this hint provide students with opportunities to engage in Math Practice 1: Make sense of problems and persevere in solving them? Students are directed to cut the remaining cookies into enough pieces for each of the students. How does this build student understanding? As a teacher, how will I know students understand how and why to partition the cookies as opposed to reading the hint? How will students take up the idea of “pieces.” Do “pieces” lead students away from fractional parts? And then there’s the bonus question that students would not be able to answer unless they have memorized DURT.
While these two pieces of instructional materials address the same standard and mathematical idea, they’re significantly different in ways that will impact student understanding.
NEXT STEPS
When looking at instructional materials, there are several questions to consider:
- What standards and math practices are addressed in each? Is the student experience aligned with the intent of the standards and math practices?
- In what ways does the task push students to develop mathematical understandings or limit students?
- How is student thinking made visible? What can I learn about student thinking?