When I first started teaching, at the end of each day, I would open my teacher’s guide, grab my pen, and thumb through the stack of completed worksheets. My eyes would dart quickly from the red answers in the teacher’s guide to the corresponding answers on each student’s page. I would dole out my x’s and checks with finality and authority. When I got to the end of a page, I would tally a percentage score and enter it into my electronic grade book. I approached every piece of student work as if it were a summative assessment.
My current approach to analyzing student work is much different. I have learned to use student work to inform my instruction. I try to think like my students and explore their reasoning. What do my students understand? Instead of looking to highlight wrong answers, I try to focus on what is right. Look at the student work below. How is Mark reasoning through these problems? What does Mark understand? Don’t focus on what he did wrong; try to articulate what he did right.
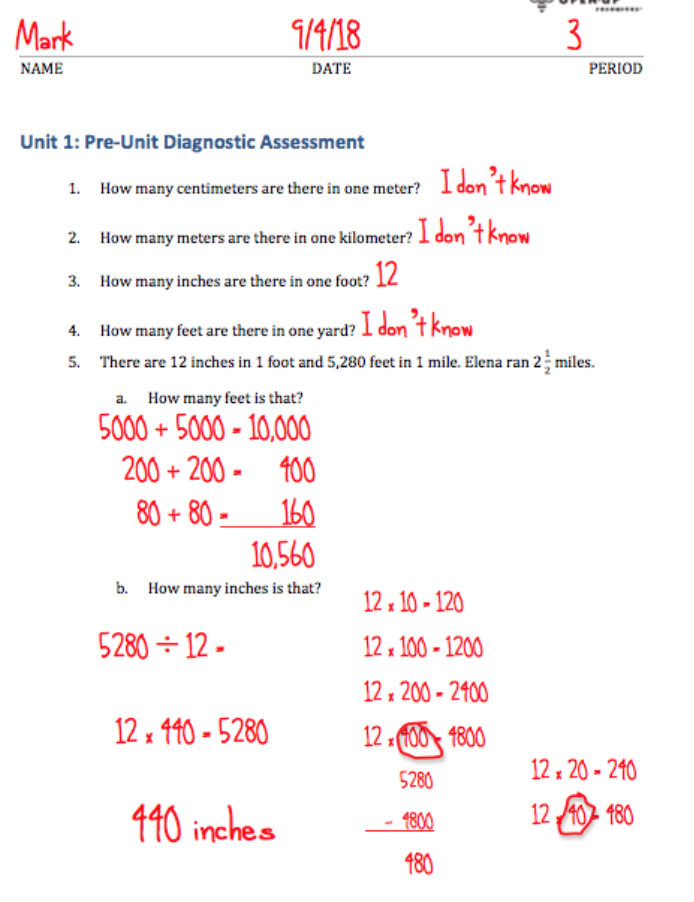
I notice that Mark:
- Doubled accurately.
- Multiplied by powers of ten.
- Used the relationship between multiplication and division to solve problems.
- Labeled his answer.
I also notice that Mark only got one question correct. If I computed a percent score for this set of problems, Mark would have answered one out of six questions correctly—a failing grade. Yet, he has demonstrated a tremendous amount of success. If I can build on them, Mark’s strategies are entry points to the thinking involved in our upcoming unit on scaling. We can use some of his ideas, like doubling, to learn more about different scale factors. Now I’m left to wonder which lessons will give Mark a chance to use his current strategies and then give the class a chance to add onto them. If Mark connects doubling to halving, then we work towards tripling and thirding.
There was a time when I would have looked at Mark’s work and felt frustrated. It would have sparked feelings of dread. I would have focused on all the things Mark did wrong. His strategies are inefficient! He can’t even multiply by ½! Does he know how to divide? His second answer isn’t reasonable! There is no way Mark is prepared for this unit. He needs intervention. He will need a lot of practice worksheets before he can even start lesson one.
Sometimes when we try to interpret student work, we make assumptions about how all the “wrong” answers will prevent students from learning grade-level material—but grade-level work rests on the foundation built from previous years. Even in wrong answers, students show glimmers of this foundational thinking.
Recently, I have been pushing myself to spend more time noticing what my students know and can do, lingering in that mindset, and postponing judgments about misconceptions and what they can’t do. This is really hard and used to feel unnatural. I have had to refine my intuition. One strategy I use is to “try on” student’s strategies—picking up a pencil and working through them myself, particularly ones that do not seem to lead to a correct solution.
Time and again, I have been joyfully surprised by the ways my students engage with math. I have learned so much about what strengths my students will bring to our classroom community. Focusing on strengths helps me be more intentional: I connect new learning to prior knowledge and orchestrate productive discussions that move learning forward for all students.
I still have a lot of work to do with Mark, and he could use some more instruction and practice with multiplying and dividing multi-digit numbers and fractions. He will also need support with unit conversion. How am I going to build on Mark’s strengths so he can access the grade-level math?
First, I’m going to review the standards that are addressed in Unit 1. Each Pre-Unit Diagnostic Assessment question is aligned to a standard. Lesson narratives contextualize the work, naming standards that the work is either “building on,” “addressing,” or “building towards,” which includes standards from earlier and later grades. I am going to read these standards and reference the course guide and other resources to help me deepen my understanding of the progression of standards that comes before and after seventh grade.
Next, I am going to look for places where Mark will have opportunities to build on his current understanding of doubling. When I look at the second lesson in Unit 1, I notice a Number Talk.
 (Click here to view the full lesson on AccessIM.org.)
(Click here to view the full lesson on AccessIM.org.)
The lesson narrative tells me that this Number Talk builds on some of the fifth-grade standards. Eventually, I will need to help Mark learn to use and understand standard algorithms, but right now I am going to try to capitalize on his understanding of doubling and help him see how it relates to halving. I am going to help him connect doubling to quadrupling, which we can then connect to finding one fourth of a whole number.
Mark may surprise me during the Number Talk. He didn’t exhibit understanding of halving on the pre-assessment, but that doesn’t mean he can’t do it, especially with a deliberate string of problems like in the Number Talk. The numbers in the problems on the pre-assessment were large, and there were multiple steps involved in solving the problems. This Number Talk will give me the opportunity to learn more about what Mark understands in regard to multiplying a fraction by a whole number.
Next Steps
Sometimes we can get so zoomed in on the grade-level math we teach that we forget to zoom out and see how it connects to the standards that come before and after our grade level. When we are too focused on what students got wrong, we overlook the information we need the most: the skills and understandings we can build on.
In order to connect new learning to prior knowledge, we have to identify what our students know and can do, which is often masked by what they are still learning. When students approach a problem that introduces new, more complex concepts, they often rely on the strategies they are most comfortable with, not necessarily the ones that are most efficient. Push yourself to postpone looking for incorrect answers and inefficient strategies. Look beyond the procedures. Find the glimmers of understanding big ideas and use them to ignite new learning.
In addition to this post, you may also enjoy:
- Inviting Students to the Math Party: Creating an Inclusive and Engaging Math Community
- The Art of Reflection

Sarah Caban
Interim Director, K–5 Teacher Learning at Illustrative Mathematics
Sarah was one of the lead authors of the Grade 5 curriculum for Illustrative Mathematics K–5 Math, and she is currently the interim director of K-5 teacher learning at Illustrative Mathematics. Sarah collaborates within and across teams to develop professional learning experiences that position teachers and students as authors of important mathematical ideas.
