“At the end of the day, this wasn’t about focusing on the objective, it was about making the objective meaningful to him.”
The work of teaching is both invigorating and challenging. We want to instill a love of math and learning in every student, and ensure that each and every child is college and career ready. How do we do this when each child’s needs are so different? How do we do this with the same set of curricular materials?
NCTM’s Principles to Actions: Ensuring Mathematical Success for All reminds us that access and equity hinge on “beliefs and practices that empower all students to participate meaningfully in learning … and to achieve outcomes in mathematics that are not predicted by or correlated with student characteristics.” (p. 60) What follows is the story of a first year teacher and two of her students. We invite you to reflect on how these curricular materials supported her in fostering strong mathematical identities in her students.
Illustrative Math 6–8 recommends that students have access to a geometry toolkit while working on related tasks. This toolkit may contain pens, colored pencils, rulers, flashcards, protractors, and—the most magical tool of all—tracing paper. Students acquaint themselves with these materials in the first unit at each grade level. As we take on different tasks, the toolkits are available for students to use as they see fit, inviting them to think about which tools are most appropriate for the task at hand.
The tracing paper is one of my favorites because it is a great tool for students to demonstrate their reasoning as they work on their written explanations. In grade 6, students can partition shapes and compare areas, in 7th grade students compare corresponding angle measures and in grade 8, students practice translations, reflections, and rotations which lead to conceptual understanding of similarity and congruence. In unit 4 of grade 8, students learn to solve systems of equations through various representations. They can balance hangers, solve algebraically, make tables or use a graph. One particular student, Charles, stood out during this unit. Throughout the year, Charles was an independent worker. He desired challenge, but waited for me to provide it. In this unit, he used the tracing paper to make a connection between a visual and a graph, and from there his curiosity pushed his thinking.
Students were given the warm-up below. What do you notice? What do you wonder?

We started by naming our two bugs—Danny Devito and Monsieur Jack Black—before proceeding with quiet think time, writing time and sharing time. After our warm-up activity students were given the following task:

Charles wondered if it was possible to connect the positions of the ladybug in the warm up to the positions of the second ladybug in the graph. I was curious, too, and encouraged him to develop his reasoning using some of the tools available (MP5: Use appropriate tools strategically). Charles asked for tracing paper and that was all he needed to go off on his own. He used the tracing paper to copy the line created by the positions of the ladybug in the warm-up and then used our chart from Unit 1 to help him determine what transformations he needed. After taking some time for trial and error, he concluded that by combining reflection and translation he could show the graph he was looking at aligned with the line he created in the warm-up.
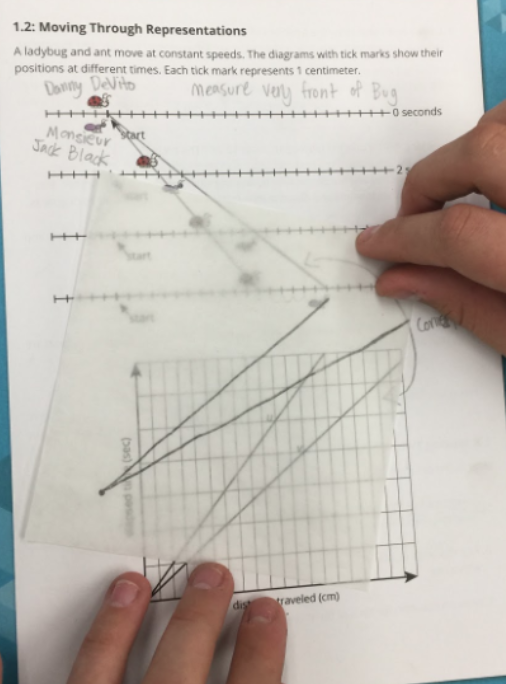
This specific moment stood out because he took initiative and ownership to determine the validity of his own argument. He didn’t just wonder and move on. He wondered and explored. All I had to do was remind him what he had available to push his own thinking. I value the opportunities students create for themselves to make connections and construct knowledge, as well as using tools to help them justify their thinking. My goal is not necessarily to have a separate activity waiting for students that are ready for more, but rather to help students develop as mathematicians. I want them to push themselves, and to pursue their own lines of thinking, like Charles did. Perhaps Charles’s mini-adventure seems unrelated to the lesson objective — but, at the end of the day, this wasn’t about focusing on the objective, it was about making the objective meaningful to him.
Meanwhile, Karen, another student, grew as a mathematician for different reasons: Karen needed time, and collaboration with other students. I could tell throughout the year that Karen was not always confident in her work. She questioned the validity of her responses and often looked for teacher confirmation to verify that her work was correct. She worked best when pushed by other students—as long as she felt comfortable with them.
By the middle of the year, I found the perfect group for Karen. The group gave her the space to think out loud and to develop her own identity. She’s an out loud thinker, she needed to be able to say “Here’s everything I’ve got,” then say “These make sense.” She wrote on the table in dry erase marker to help her peers understand what she was trying to say. She wasn’t afraid to try something else and admit “This didn’t work. Ok! What else can we try?” This group often engaged in group discussion and they fed off each other’s ways of thinking to determine their answers. If they agreed, they had more than one way of showing it and if they didn’t they discussed why their answers differed all while making sure they communicated clearly and efficiently by using the academic language of our class. I was so impressed by how much this group could do and I could see that Karen was not scared to disagree with someone or ask for a teammates help to show her own thinking using a different method.

As the year progressed, Karen was not afraid to raise her hand to share or correct a peer’s thinking, even outside her group. By the end of the year, she could lead the class through a problem by showing her work, describing her process, and accepting any feedback her peers offered her. I was so proud of how far she had come and it was truly beautiful to see her shine in that moment. During one of the last days of school I saw her by our school garden and I paused to say good morning. That’s when I asked her, “What’s your history with math?” She was honest and said it was not always the easiest for her but that “math [was] different this year.”
Math. Different. Different for her, her teacher, and for her peers. This was one teacher’s story of how she built students’ sense of agency and identity, with this curriculum as her vehicle.
Next Step
NCTM’s Principles to Action calls for educators to “develop socially, emotionally, and academically safe environments for mathematics teaching and learning—environments in which students feel safe to engage with one another and with teachers.” (p.115) We’re curious to learn what you are doing. Please share your stories, pictures, blog post links, and reflections in the comments section below, or on Twitter using our #LearnWithIM hashtag and tagging @IllustrateMath!