By Melissa Schumacher, Curriculum Writer
Which is more important for students to have: conceptual understanding or procedural fluency? Does one have to be taught before the other can emerge?
Some argue that procedure has to come first, because without procedural fluency, students won’t be able to do the work they need to notice patterns. I’ve seen students who were more procedurally fluent develop deeper conceptual understanding because they didn’t get bogged down in calculations. But I had always believed that conceptual understanding was ultimately more important, because procedural fluency is sterile and fragile without the underlying knowledge of how and why the procedures work. I thought procedures shouldn’t be taught without at least giving students some idea of why they work first.
Now I see that conceptual understanding and procedural fluency can be developed together. This is embodied in IM’s design principle, Developing Conceptual Understanding and Procedural Fluency: “As the unit progresses, students are systematically introduced to representations, contexts, concepts, language and notation. As their learning progresses, they make connections between different representations and strategies, consolidating their conceptual understanding, and see and understand more efficient methods of solving problems, supporting the shift towards procedural fluency.” Conceptual and procedural work are interleaved throughout each unit. But they can also be combined in single activities, and I think this is where the rubber really hits the road.
As a curriculum writer for IM Algebra 2, this design principle guides me to write activities that involve both conceptual and procedural work whenever possible. One way is to ask students to use conceptual understanding to make a prediction, and then check their answer by doing a procedure. This activity from our Algebra 2 unit on polynomials is a great example:
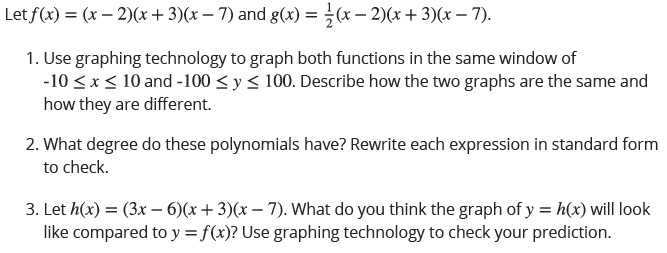
On the surface, this is a relatively straightforward activity making a simple point: that functions can have the same zeros, but look different because they’re scaled differently. However, in doing this activity, students do much more than learn about the effect of a scale factor. They use graphing technology to notice differences between functions—why are they different? They figure out the degrees using their developing conceptual understanding of polynomials, and check their answer themselves procedurally by multiplying out the factors. They make a prediction about a new graph based on their understanding of the examples they’ve seen, and they check that prediction too. Along the way, students are invited into the mathematical work, have the freedom to choose how they do that work, and are empowered to evaluate the results on their own. Through activities like this, conceptual understanding is supported by procedural fluency, and vice versa.
Next Steps
So break down the barriers between conceptual understanding and procedural fluency! Look for ways to promote both at once. It’s not a zero-sum game: you don’t have to take a day out of teaching to give students drill-and-kill worksheets, and students don’t need to understand why a certain shortcut works before they see problems that would usually be best solved with the shortcut. Let them struggle (productively) and find shortcuts on their own. Ask them to check their own work using another method rather than relying on answers from you, and ask them to justify the procedural steps they used and to look for more efficient or reliable methods.
In short, conceptual understanding and procedural fluency are two good things that go better together.