By Kristin Gray
This was originally posted on Kristin Gray’s personal blog, Math Minds, on November 15, 2020.
Student work is just the best. It is the one thing that will always motivate me to write! So, let’s kick this post off with a great work example from grade 3. In this task, students are asked to locate 1 on a number line labeled with 0 and $\frac{1}{3}$.

When I look at student work, I typically think about 3 things:
- I look for evidence of what the student understands. In this case, it also involves making some assumptions because we can only know so much based on written work.
- I think about question(s) I would want to ask the student. Again, because we can only know so much from written work, there can be so many hidden understandings that can only be found by talking with the student.
- I think about next steps, in particular, how I might create a connected, grade-level onramp experience that might be helpful for the student.
Before reading on, what do you see in the work above? Take a minute to jot down your thoughts. I would love for you to share your ideas in the comment section!
Here are some ideas I think the student understands based on the work:
- Because the denominators increase from 1 to 5, there is evidence of an understanding that whole numbers increase as they move to the right on the number line.
- Because the unit fractions are all between 0 and 1, there is evidence the student knows that unit fractions are less than 1 and can be in many denominations, especially since 5ths are not typically in the work of earlier grades.
- Because the fractions are spaced out fairly equally on the number line, there is evidence the student understands there should be equal intervals between numbers on a number line.
These are great understandings on which to build for a problem like this. So, what is up with those denominators increasing to the right?
At first, I thought the student was counting the denominator like whole numbers and thinking fractions are always written with a 1 above the fraction line, so it was just “put there” but had no meaning. But then, in the same set of student work, I saw this cool-down.

This student did a similar thing with the increasing denominators, but it was the sketch in the corner that caught my eye. This student is showing the number of equal parts in a whole for halves and fourths. This made me wonder if the denominator did have a fractional meaning to them and not just a counting number in a sequence.
If the student understands the denominator as a number that represents the number of equal parts in a whole, then it makes total sense that they would label them in increasing order. In that way of thinking, the denominator is like a whole number in their mind and whole numbers increase to the right on the number line. For example halves are 2 parts, thirds are 3 parts, fourths are 4 parts, etc. So the more parts, the larger the fraction in their eyes. They see the fraction as a numerator and denominator, 2 numbers with different meanings, rather than 1 number located on the number line.
As I looked back at the progression, I realized how intricate this move is from K–2 understanding of fractions as a quantity to grade 3 understanding of fractions as numbers.
Using the Standards as a reference, in grade 2 students use fraction language to describe quantities such as “half of a rectangle” or “a fourth of a circle.” That half and fourth are quantities that can be a different size each time depending on the whole because it is a fraction of a shape. At the same grade level, within their measurement work, students are locating whole numbers on a number line.
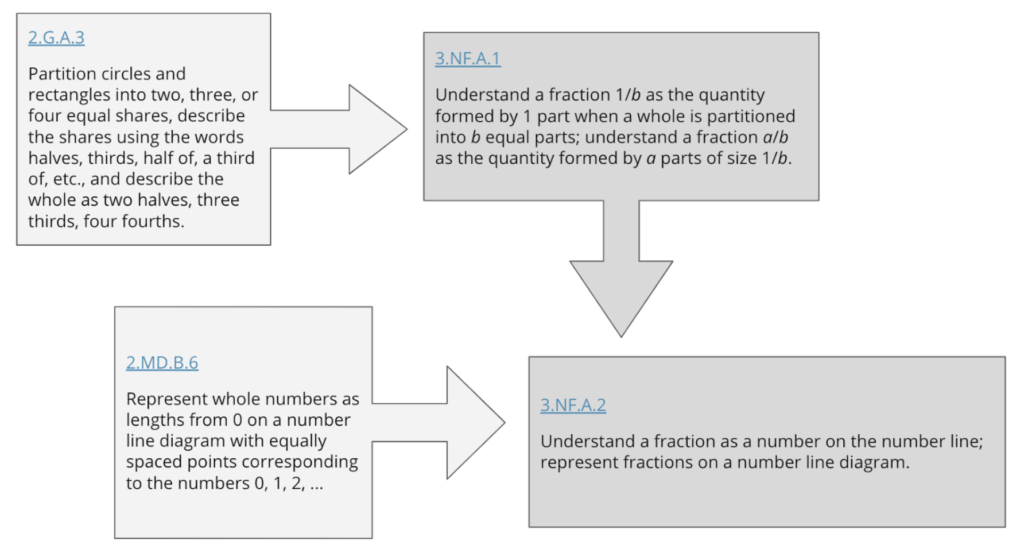
In grade 3, students learn fraction notation to represent those quantities and later merge that understanding with the early number line work to understand a fraction as a number on the number line. That number has a specific location and does not vary in the way the quantities did in earlier grades. It is a super intricate move in fraction understanding which makes it easy to understand why students have brilliant moments like the one in the cool-downs above.
So, let’s revisit the student work.
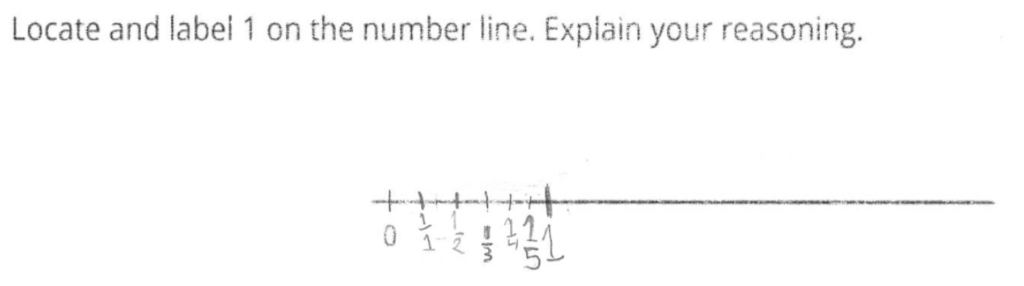
To learn more about their thinking, I would love to ask the following questions:
- How did you use the $\frac{1}{3}$ to locate the other fractions?
- In this question, I am curious if the student split the distance from 0–$\frac{1}{3}$ into 3 parts (bc of the 3 in thirds) and then did the same on the other side of $\frac{1}{3}$ and that is how they determined to stop at $\frac{1}{5}$ and then put 1 – three intervals on each side of the $\frac{1}{3}$.
- What did you pay attention to when you put the other fractions on the number line?
- I want to hear about the equal spacing of the fractions.
- Why did you stop at $\frac{1}{5}$? Where would you put $\frac{1}{6}$?
- This could be answered in question 1, but if not, I am so curious about $\frac{1}{6}$.
- [draw a number line labeled with 0–1] Where is $\frac{1}{2}$?
- Do they know $\frac{1}{2}$ is in the middle of 0–1?
- [draw an identical number line below the first one] Where is $\frac{1}{3}$?
- Do they know it is 3 equal intervals between 0–1? And, if the number lines are the same length, I want to see if they can reason about $\frac{1}{2}$ being larger than $\frac{1}{3}$.
Now, the fun part of thinking about next steps. How can we connect what students understand about equal-sized parts to understanding a fraction as a number on the number line? It seems like activities that connect the number of parts in a whole to the size of one part would be helpful here. Right now, I think the students understand $\frac{1}{4}$ as 4 parts and maybe $\frac{3}{4}$ as 3 out of 4 parts. But, when moving to understanding a fraction as a number we want them to understand $\frac{1}{4}$ as the size of 1 part of a whole partitioned into 4 equal parts and $\frac{3}{4}$ as 3 pieces the size of $\frac{1}{4}$.
There are many ways to think about this but below is a sequence of learning that I think would be nice.
- To connect to the 2.G.A.3 work, we could:
- Have several same-sized rectangles drawn one above another. Ask students to divide the top one into halves, the middle one into thirds, and the bottom one into fourths. (grade 2 work)
- Ask them how they would name each piece and what they notice about the size of the pieces. (grade 2 work)
- Ask them how they would record the size of each piece. If they use fraction notation that is grade 3 work.
- Transition to creating fraction strips for halves, thirds, and fourths. Label the fractional pieces of the strips and discuss the size of the pieces and the number of pieces in the whole. (The fraction strips from Marilyn’s Do the Math kits made a world of difference in my 5th graders understanding of fractions!)
- Place the fraction strips above a number line labeled with 0 and 1.
- Ask students where $\frac{1}{2}$, $\frac{1}{3}$, and $\frac{1}{4}$ would be located on the number line based on what they know about the length of the fraction strips.
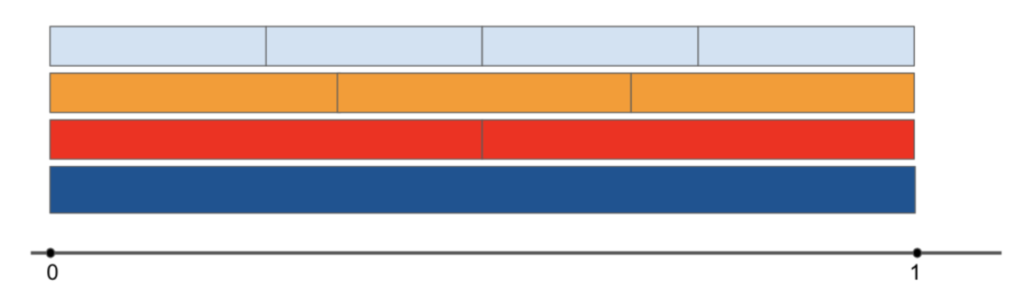
- Ask where they think non-unit fractions might go? Where is $\frac{2}{3}$? Where is $\frac{2}{4}$? Where is $\frac{3}{4}$?
- Ask for different ways they could label 1.
Then, I would have them revisit the cool-down and see what they do! There are also more of my favorite number line tasks from IM here. Try some out and dig into the student thinking. It is always such an amazing learning experience!
Next Steps
Try examining some student work with these questions in mind:
- How do you frame your thinking about student work?
- What questions would you want to ask the student?
- How can examining the learning progressions help position the students’ current understanding and where you want to nudge them next?