by Joe Herbert
“These problems are great, but they’re just so wordy. My students can’t handle all that reading!”
Does this feel familiar? If so, you’re not alone! Many students and teachers struggle with the language demands of IM 6–12 Math when they first start their implementation journey.
Students may not be used to the level of reading and writing in math that this curriculum requires, and teachers may be unsure of how to support students with these literacy demands, particularly if their students are more comfortable in a language other than English.
Luckily, the curriculum authors have integrated eight Mathematical Language Routines (MLRs)* into the curriculum to support teachers in amplifying, assessing, and developing students’ language.
While these routines are specifically tagged as supporting Access for English Learners, they are actually beneficial to all learners.
One of the core tenets of language support in this curriculum is that we amplify rather than simplify language. I’m going to be honest: when I first heard the phrase “amplify rather than simplify,” I was confused by what it meant. But by digging into one of the Mathematical Language Routines— MLR6: Three Reads**—I finally understood the philosophy, and why it is so powerful in making sure that all students have access to mathematics, while keeping the focus on authentic sense-making.
In this blog post, I’m going to walk through the mechanics of implementing MLR6: Three Reads using an example from the curriculum. I’ll also share some anecdotes and tips from my experience facilitating this routine—both in a GenEd classroom, and in a sheltered class dedicated to newcomers who are just beginning to learn and use English.
While I’m going to illustrate this routine using a high school example, this same routine structure can be applied to middle school and even elementary school contexts.
The example we’ll look at in this post comes from Geometry Unit 5 Lesson 17. I think it’s always helpful to know where students are in their learning trajectory, especially when looking at an example that comes so late in a unit. Before this lesson, students have thought deeply about volume, and know how to find the volume of many solids. Early in this lesson, they’re introduced to the concept of density as an application of volume.
With that in mind, here is the activity:
An aquarium manager drew a blueprint for a cylindrical fish tank. The tank has a vertical tube in the middle in which visitors can stand and view the fish.
The best average density for the species of fish that will go in the tank is 16 fish per 100 gallons of water. This provides enough room for the fish to swim while making sure that there are plenty of fish for people to see.
The aquarium has 275 fish available to put in the tank. Is this the right number of fish for the tank? If not, how many fish should be added or removed? Explain your reasoning.
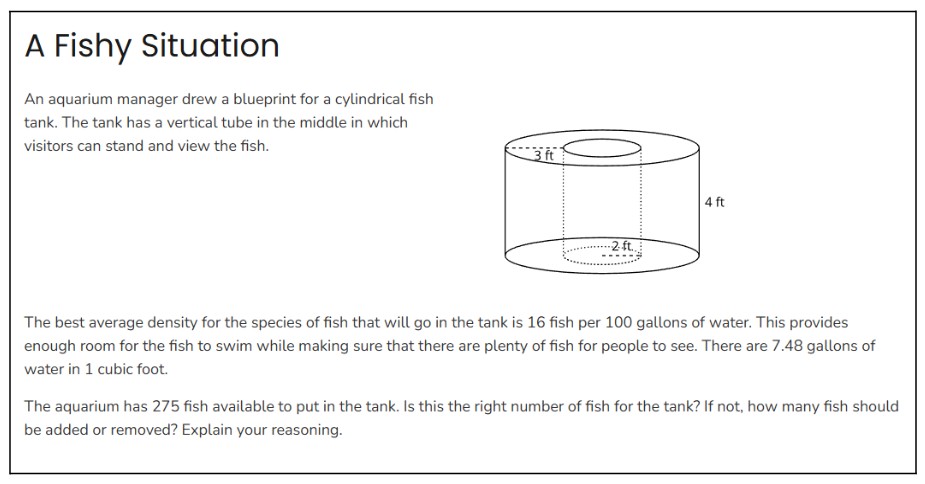
If your head is spinning from all of the information you’re trying to keep track of in this problem, you’re not alone! Every teacher can probably think of at least one student (and maybe a whole class of students!) who would get intimidated by this problem and not know where to start.
That’s where MLR6: Three Reads comes into play. Rather than simplifying the problem and removing some of the academic rigor, Three Reads provides a structured format for teachers and students to partner in amplifying the language in the problem, so that students are able to understand it and engage in authentic sense-making.
Let’s dig into how the routine does this. As the name suggests, in the Three Reads routine, we engage in three structured readings of the same problem context, with a slightly different and more focused lens each time.
Read 1: Understand the Story Context
In the first read, the goal is just to understand the context of the problem.
To help students focus solely on the story context, we remove the question. Thus, in our example, we would just share the following with students:
An aquarium manager drew a blueprint for a cylindrical fish tank. The tank has a vertical tube in the middle in which visitors can stand and view the fish.
The best average density for the species of fish that will go in the tank is 16 fish per 100 gallons of water. This provides enough room for the fish to swim while making sure that there are plenty of fish for people to see.
The aquarium has 275 fish available to put in the tank.
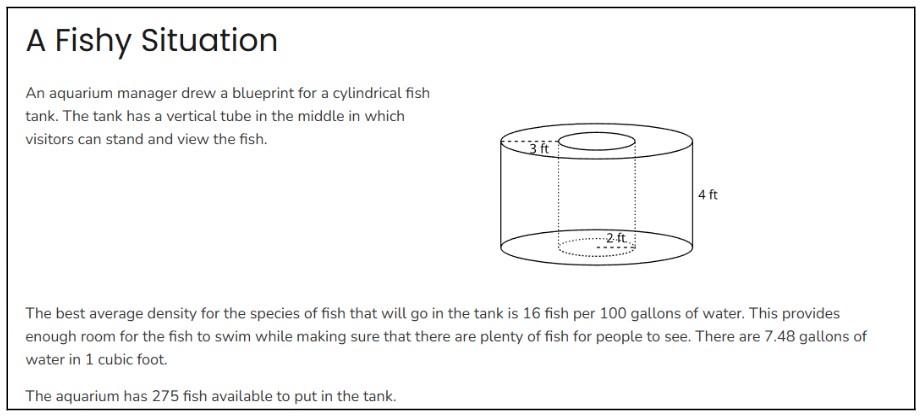
When doing this first read, I often read out loud to the class. Alternatively, you can have a student read out loud. If you teach English Learners, this is the time to bring in visual aids, such as pictures from your local aquarium.
The discussion that follows Read 1 has a very clear goal: make sure students understand the story context of the problem, and address any non-mathematical vocabulary words that might get in students’ way.
After the shared reading, we ask students “What is this situation about?” or “What is the story of this problem?”
Then, scribe their ideas on the whiteboard or some other shared space.
Students sometimes start jumping to talking about the numbers that are in the problem, but I specifically ask them to tell me what the story of the problem is without referencing any numbers at all. By the end of this discussion, I want to make sure students know what a fish tank is, and that they recognize the unique shape of the particular fish tank in this problem. I might even draw a little stick figure in the inner cylinder to really drive the point home.
Read 2: Identify Quantities
The second time we read the problem together, we again omit the question so that students can focus exclusively on making sense of the context. The mechanics for how I implement Read 2 sometimes differ depending on the audience.
With newcomers who are just learning how to read (and speak!) in English, I like to do a choral read aloud. This gives students a low-stakes opportunity to practice reading in English, because everyone is reading at the same time so no one individual voice can be easily heard.
In my experience, in a GenEd classroom, high schoolers prefer to read the problem together in small groups, rather than choral reading.
Now for the important part: the questions we ask and discussion we have after Read 2.
Here is where we ask students, “What can be counted or measured?”, without focusing on any specific numbers. In our Geometry example, this might include the volume of the tank, the density of fish, the dimensions of the tank, and the number of fish available.
When working with newcomer English learners, I have had some trouble explaining what I mean by the question “What can be counted or measured?”. In that case, I modify the routine slightly to ask students what quantities they see in the problem, and I specify that a quantity is a number and its unit***. Thus, rather than just identifying the number 3, for example, students will identify that the distance between the inner and outer cylinders is 3 feet.
Regardless of the specific prompt you give students, the main goal for Read 2 is for students to begin to think more deeply about the various quantities in the problem, how they’re related to each other, and how they relate to the overall story context. As in Read 1, we scribe students’ ideas on the whiteboard to help make our collective thinking visible.
Read 3: Reveal the Questions and Plan Solution Strategies
Read 3 is where we read the context one last time, and then reveal the actual questions students will investigate.
The mechanics for Read 3 can be whatever works best for your students: choral reading, reading in small groups, or one volunteer reading out loud.
After the shared reading, the discussion prompt for Read 3 is, “What are some ways we might solve this?” The goal is for students to do some high-level planning and strategizing before they jump into the nitty gritty of computation.
In our example, we might have students who talk about finding the total volume of the big cylinder, and then subtracting the volume of the smaller cylinder. Alternatively, they might discuss finding the area of the base of the fish tank and multiplying it by the height to find the volume.
By discussing these ideas and strategies in partner pairs or as a whole class, we set kids up for success when we release them to independent or group work.
Amplify, Don’t Simplify
I hope this breakdown of MLR6: Three Reads makes clear how we can use Mathematical Language Routines to amplify rather than simplify language. By engaging in this routine, we’re not changing the wording of the problem to make it simpler. Rather, we’re using the three structured reads and discussion prompts to amplify the language in the problem and help students make sense of the problem for themselves.
The first time I tried Three Reads in the classroom, I had several students who had arrived in the US earlier that week. They were just starting to learn English, and did not feel very comfortable with it yet. However, after engaging in the routine, they made sense of the problems authentically, and offered meaningful contributions to their groups. (I of course encouraged students to discuss the problems with peers in whatever language they felt most comfortable with.)
This was when I truly understood the incredible power of this routine to help create a world where all learners know, use, and enjoy mathematics.
I hope that this leaves you eager to try MLR6: Three Reads in your classroom, regardless of the student population you teach.
If you’re feeling excited but still a little intimidated, I’d like to draw your attention to the Illustrative Mathematics teacher notes. I think it’s helpful to see how much support the curriculum authors have given teachers in making IM 6–12 Math accessible to all.
This is the suggestion to support Access for English Learners for the fish tank activity:
Notice how this suggestion goes beyond just saying, “do Three Reads,” and instead highlights the important ideas that you want to draw out of the conversation after each of the three reads.
Does MLR6: Three Reads take some extra instructional time? Yes, of course. However, if your students are able to dive straightaway into authentic sense-making after engaging in the routine, then it is time well spent.
Next Steps
So the next time you get overwhelmed thinking about how your students are going to struggle with a wordy problem, take a look at the suggestions for access that are built into the curriculum. Whether or not you teach English learners, you might find a suggestion for a Mathematical Language Routine that provides the perfect support to help you amplify rather than simplify language for your students!
*Zwiers, J., Dieckmann, J., Rutherford-Quach, S., Daro, V., Skarin, R., Weiss, S., & Malamut, J. (2017). Principles for the Design of Mathematics Curricula: Promoting Language and Content Development
**Kelemanik, G, Lucenta, A & Creighton, S.J. (2016). Routines for reasoning: Fostering the mathematical practices in all students. Portsmouth, NH: Heinemann.
*** This modification for Read 2 comes from the Three Reads protocol distributed by San Francisco Unified School District
