By Cheryl Fricchione, IMCF and Implementation Development Team Member
A quick Google search for “kindergarten math centers” produces over 20 million results: everything from tips to make centers work to theme-based printables. It brings to mind a vignette about an annual fall unit on apples, from the introduction to Grant Wiggins and Jay McTighe’s Understanding by Design. Theme-based printables are often engaging for students, but do not always have clear learning goals, and typically have not undergone thorough review to ensure standard-alignment.
What Makes IM’s Centers Different?
Center stages in IM are always aligned to Common Core standards. These centers deliberately build mathematical ideas and understandings, and often feature multiple stages that support coherent learning progressions. In addition, as the IM Course Guide says: In kindergarten, “center time is built into lessons so that students have a chance to spend more time on topics that require more time to develop understanding”.
For example, students are first introduced to “Pattern Blocks, Stage 1: Explore in Unit 1, Lesson 2“. This stage gives students the opportunity “to explore and use,” or play with, pattern blocks as they build towards the following standards:
- K.CC.B: Count to tell the number of objects.
- K.MD.B.3: Classify objects into given categories; count the numbers of objects in each category and sort the categories by count. Limit category counts to be less than or equal to 10.
Centers as Authentic Assessment
Most educators agree on the importance of play in early childhood classrooms. Often, though, there is a misguided belief that these classrooms must choose between play and mathematics. However, research by Seo and Ginsburg has shown that typically young children engage in mathematical thinking at least once every 30 seconds that they are playing.
Therefore, as students engage with centers—even ones that allow for free exploration—teachers have opportunities to authentically assess student mathematical thinking. The center mentioned earlier, “Pattern Blocks, Stage 1: Explore” specifically offers teachers the chance to learn more about students’ counting concepts and skills. The lesson narrative (Unit 1, Lesson 2) provides questions teachers can ask to formatively assess mathematical thinking, including:
- How many red pattern blocks do you have in your object?
- How many pattern blocks are on the bottom of your object?
Centers and Section Checklists
When I talk to educators using IM K–5 Math, I often say that centers are not like a crock pot: you cannot simply set them and forget them. To get the most out of the experience, it is important for teachers to regularly engage with students as they play at centers.
Diagnostic interviews and written assessments in kindergarten typically reduce instruction time. Teachers tell us it is not unusual to take a full day for each section checklist. Beth L. MacDonald and Jessica F. Shumway have said that using game play for ongoing formative assessments can “immediately inform educators of appropriate instructional strategies without stopping direct instruction.” Students are able to learn and practice while they play, and teachers can use section checklists to inform how teachers observe and confer with students. Let’s consider how Section Checklists support assessment during center time.
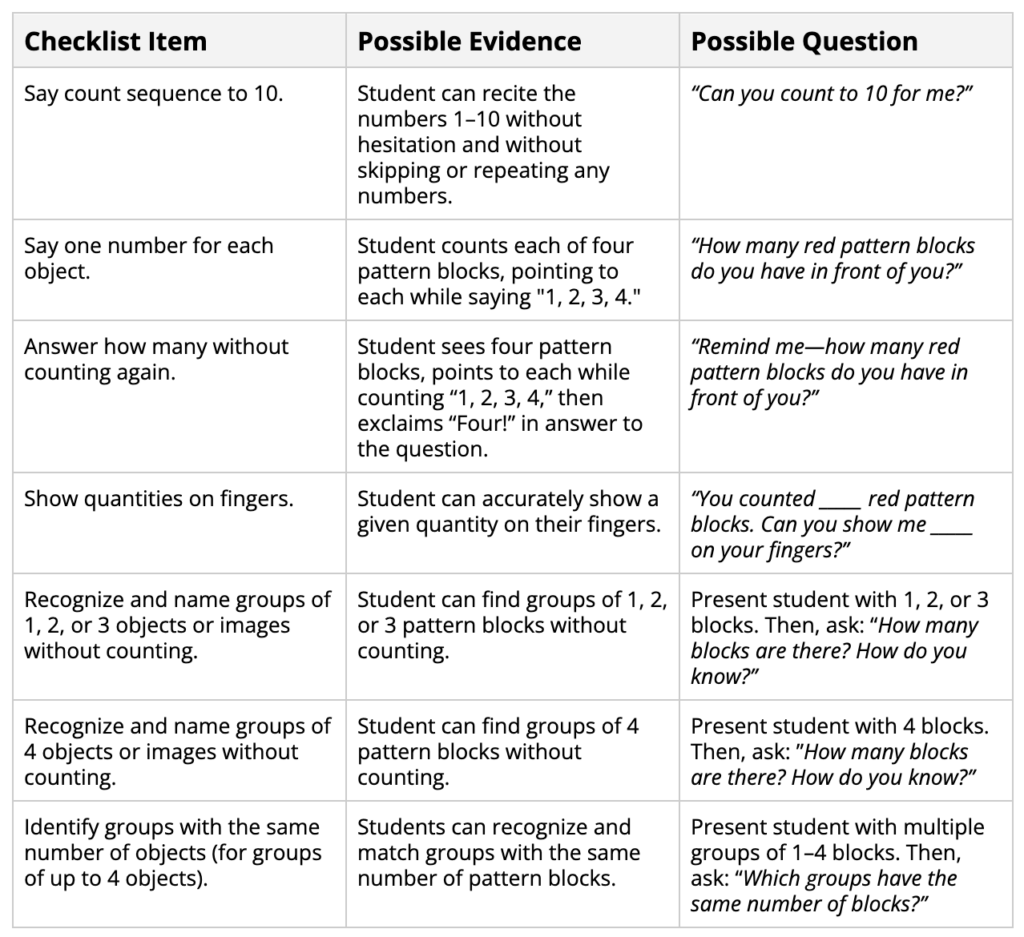
The items on Kindergarten’s Unit 1 Section Checklist for all Sections A–D include:
- Say the count sequence to 10.
- Say one number for each object.
- Answer how many without counting again.
- Show quantities on fingers.
- Recognize and name groups of 1, 2, or 3 objects or images without counting.
- Recognize and name groups of 4 objects or images without counting.
- Identify groups with the same number of objects (for groups of up to 4 objects).
To start, we want to consider what evidence of learning for each of these items might be found as students play “Pattern Blocks, Stage 1: Explore.” Next, we want to determine what questions teachers might ask to assess student understanding. Finally, we want to determine what components (if any) might not be able to be assessed as students engage with this particular center.
This table shows an example of how you might monitor student progress towards the items on the Unit 1 Section Checklist during “Pattern Blocks, Stage 1: Explore”:
Let’s Practice
At the beginning of the school year I had the pleasure of chatting with some kindergarteners as they engaged in “Pattern Blocks, Stage 1: Explore.“
I asked them many of the questions in the table above. Let’s focus on the second item on the checklist: Say one number for each object. Note that in some of the videos students are asked to count between 6–10 objects. These students had already successfully demonstrated one-to-one correspondence with up to 5 objects and while not required for mastery during Unit 1, counting up to 10 objects supports students in Unit 2, which focuses more deeply on numbers 1–10. For each student, let’s consider whether we have evidence the student has demonstrated the skill (✓), we do not have evidence the student has demonstrated the skill (✗), or we are unsure at this time and would like additional evidence (?). Let’s also consider if we have evidence of any other items on the checklist.
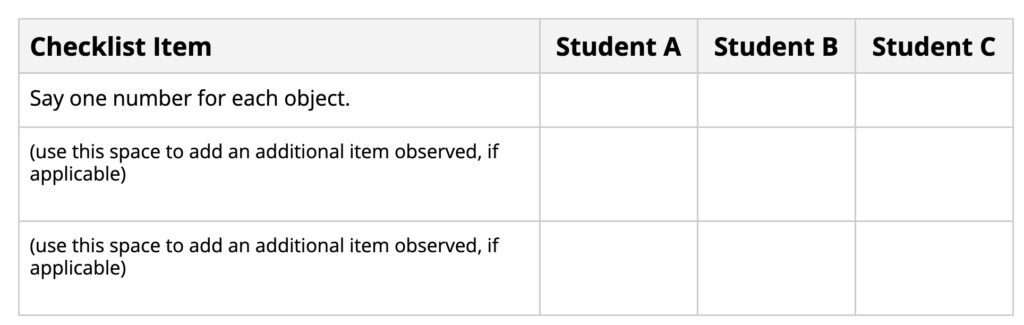
| STUDENT A | STUDENT B | STUDENT C |
Additional Considerations
You may be wondering how teachers can find the time to talk to every student during centers. It is important to note that many centers are used across multiple lessons. For example, after being introduced in Lesson 2 of Unit 1, “Pattern Blocks” (in various stages) appears as a center choice in Lessons 5 through 16 of the unit. This means that teachers do not need to try to talk to every student every day. Instead, teachers can create a rotating schedule. For example, if a classroom has 25 students the teacher may split them into five groups of five and talk to the students in one group each day. It is also important to remember that once teachers have clear and convincing evidence that a student has demonstrated a particular skill they do not need to assess the skill again even if it is listed as an observation in a later lesson.
Conclusion
Not all centers are created equal. While centers can be a playful experience, there is a difference between students just rolling a number cube or moving a marker to “win” and playing a game while also consolidating and applying their learning. As the Course Guide states, two key purposes of IM centers are to “build towards the content in a lesson or section” and “develop fluency across that grade level”. Accordingly, IM centers allow teachers an authentic time to be curious about and collect student thinking, and to confer with students as assessment. Teachers can use the information they gain by observing students at centers to plan for and respond to student thinking in future lessons.
Next Steps
- Now that you have watched the videos, share your thoughts on this Jamboard. Where do you think each of these students stands in their progress towards the Unit 1 learning goals? What might you plan to do next with each of them? (The first few frames focus on student’ ability to say one number for each object. The remaining frames offer an opportunity to comment on any other items observed.)
- Download this PDF of the Center Assessment Planning document, pick a center from your upcoming unit and give it a try yourself!
- Share your ahas, ideas, and plans with us on Twitter using #LearnwithIM.
References
McTighe, Jay and Grant P. Wiggins. (2004). Understanding by Design : Professional Development Workbook. Alexandria, Va.: Association for Supervision and Curriculum Development.
MacDonald, Beth L. & Jessica F. Shumway. (2016). Subitizing games: Assessing preschoolers’ number understanding: Reflect and discuss. Teaching Children Mathematics, 22(6), 340–348.
Seo, K.-H., & Ginsburg, H. P. (2004). What is developmentally appropriate in early childhood mathematics education? In D. H. Clements, J. Sarama, & A.-M. DiBiase (Eds.), Engaging young children in mathematics: Standards for early childhood mathematics education (pp. 91-104). Mahwah, NJ: Erlbaum.
