By Greta Anderson, CEO of Greta Anderson Consulting, LLC and IM Certified Facilitator
There’s a common misconception that has surfaced on a checkpoint, but no additional minutes or days to reteach. How will this impact implementation and pacing? How in the world are teachers supposed to proceed? Stop and risk not covering a full year’s content every time this happens? Or go forward and hope that students take it upon themselves to shore up the areas where they had confusion? Neither of these options are good for students, but there is a third path: leveraging future content to address misconceptions.
Over the past several years, I have partnered with schools on their IM implementation journeys, and the scenario described above is quite common. Because I have worked with the IM curriculum for many years, I have a bit more context on the direction of the curriculum than first-year implementers and try to help teams see where there are natural places to respond to misconceptions. In this blog post, I am sharing a specific scenario that might benefit schools in their own data analysis.
I work with a fourth-grade team that expressed concern about student performance on the Grade 4 Unit 2 Section A checkpoint. I met with the team to think about how to respond. We began by setting some norms about how we agree to approach this conversation:
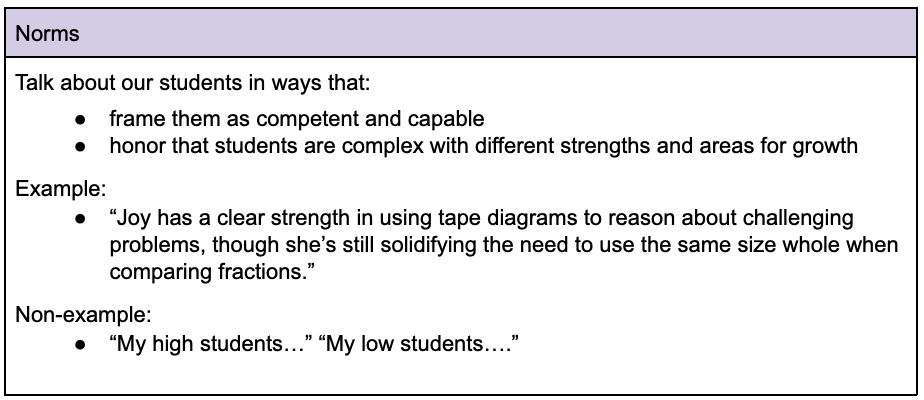
We discussed how and why the example and non-examples affirmed or undermined our norms, respectively. These norms were especially relevant given that things like grade-level equivalencies can be misleading—many of the current fourth-grade students had severely disrupted first- and second-grade experiences, which may result in emerging understanding of addition and subtraction using place-value strategies. That said, many third-grade students from this year had a more normal experience and might have full understanding of third-grade fraction standards, especially since the third-grade fraction standards do not require operating with fractions. In short, we want to ground in what students do understand. Blanket labels such as “high,” “low,” and “bubble kids” do not honor our students as competent, capable, and complex. They distract us from the ever-pressing work of examining what students understand about the current content.
We then looked through a class set of student work on this checkpoint and thought about the different understandings this checkpoint might reveal:
- locating fractions on a number line when the whole is given
- locating fractions on a number line when the whole is not given
- explaining why a fraction is more/less than half
- explaining why two fractions are equivalent
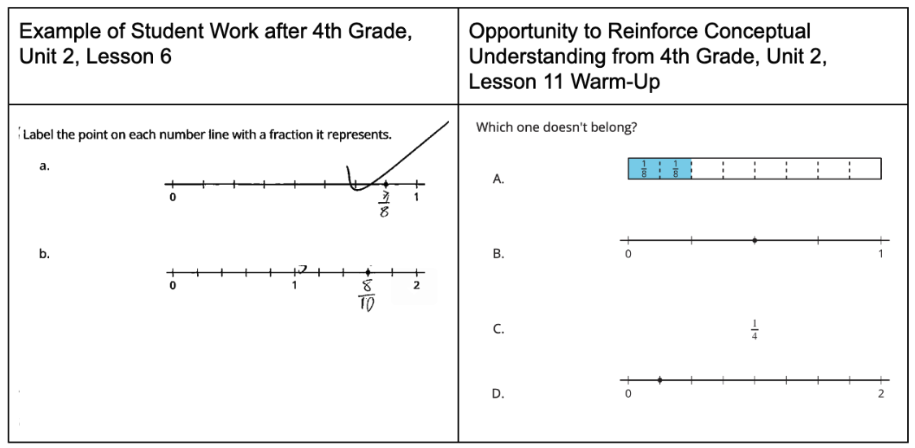
We knew that this is the Section A checkpoint and that Section B goes much deeper on equivalency, so we thought the first two bullet points might be the place to focus our attention for now. We looked across student work and noticed a common misconception where many students were successful in noticing how many equal parts were present, but did not always consider that we should think about the number of equal parts making up 1 whole.
Instead of carving out time to reteach, we thought about looking for future opportunities to reinforce conceptual understanding. We saw a great opportunity several lessons ahead to revisit this concept. Although there were other opportunities before then, this Which One Doesn’t Belong felt like an accessible entry point for additional practice!
We thought about what opportunities there were to address some of the misconceptions from the checkpoint. Here is where we landed:
- Facilitate the routine as intended, but take fewer ideas about A, B, and C to allow time for a deeper conversation about D since that represents a common misconception.
- Plan for a conversation about D that seeks out a student willing to share why they see $\frac18$ as the value shown on the number line and a student willing to share why they see ¼ as the value shown on the number line. Validate that students seeing $\frac18$ are noticing 8 equal parts (great!) while also eliciting the idea from students that $\frac14$ establishes the number of equal parts in 1 whole. Be intentional and write this idea clearly on the chart paper when it is named!
- Ensure that the visuals and ideas are captured on chart paper so that we can keep this up as a reference for students as they solidify their understanding. Encourage students to use this resource in the future as well.
- We also noted other facilitation opportunities that were transferable across future lessons, such as using choral counts, writing in unit form to establish the denominator as the unit size and the numerator as the count, and having students annotate helpful student strategies in their workbooks.
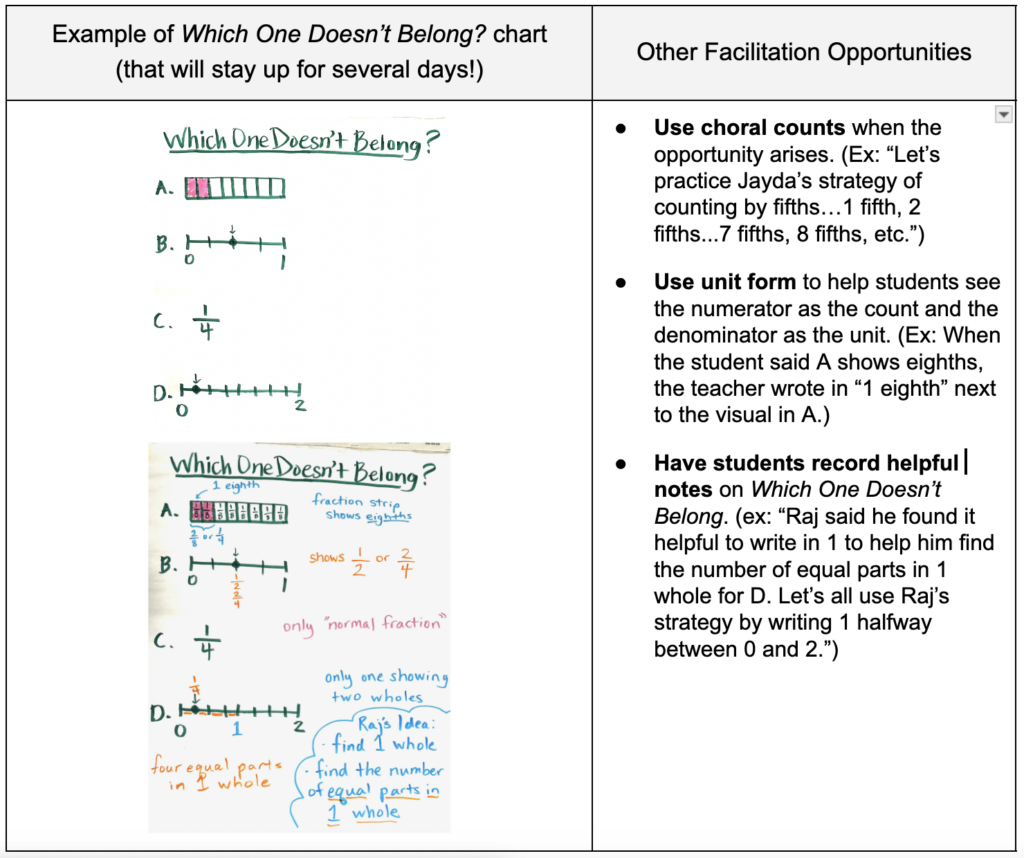
As teachers continue their IM journey, I encourage them to note areas where they’d like to focus more attention and check out upcoming warm-ups before making a plan to reteach. There are often opportunities to build and solidify conceptual understanding. Leveraging semi-permanent anchor charts with students’ noticings and wonderings, connections across mathematical ideas, or surfaced vocabulary, provides a resource for students and promotes community while enabling the teacher to give access points for all students.
Next Steps
As you move along your implementation journey and experience moments of, “Oh no, I don’t think they’ve got it!,” resist the urge to assume that students can’t access the grade-level content. Try these suggestions instead:
- Look at the student work from the most recent checkpoint. (Kindergarten teachers should look at the trends in their observation checklist.)
- Look ahead at the warm-ups in the remainder of the unit.
- Identify areas to prioritize for extra clarity.
- Plan for semi-permanent displays if possible.
 Greta Anderson, CEO of Greta Anderson Consulting, LLC and IM Certified Facilitator
Greta Anderson, CEO of Greta Anderson Consulting, LLC and IM Certified Facilitator
Greta has been teaching and supporting mathematics in New Orleans, Louisiana, since 2005 and has an M.Ed. in instructional leadership in mathematics. Greta has also worked on the Illustrative Mathematics team as a task writer and reviewer since 2012, and as an IM Certified Facilitator since 2017. She works directly with schools to support IM curriculum implementation. Greta is an alumna of Park City Mathematics Institute and the Dana Center’s International Facilitation Fellowship. She loves running, beach volleyball, and snuggles with her dog.
