by Tina Cardone
The vision of Illustrative Mathematics is to create a world where learners know, use, and enjoy mathematics. This raises the question: Which learners? And what role do the authors of a curriculum play in shaping the experience?
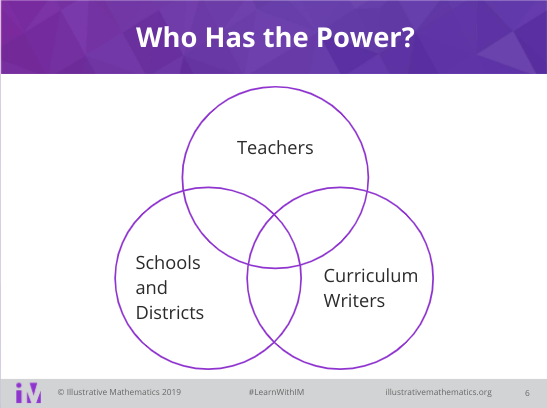
There are multiple spheres of influence that impact the experiences students have in their math classrooms. And each of those spheres has some influence over the others as well as over the students. The authors of IM K–12 Math think about equity—how it can shape structural decisions at a school level (or broader), and how it can shape experiences at a classroom level. This post will focus on the classroom level of change.
One way to check for equity in a classroom or curriculum is through the effective teaching practices. NCTM’s Catalyzing Change (2018) challenges decision makers in math education to make shifts that promote equity. In a previous post, we discussed how IM’s curricula support three critical calls to action identified in the book: ending tracking, implementing effective targeted instructional supports, and broadening the focus of teaching high school mathematics beyond college and career readiness. The book also calls for implementing equitable instruction. Catalyzing Change defines equitable instruction using a crosswalk from the 8 mathematics teaching practices (from NCTM’s Principles to Action) to additional equitable teaching criteria aligned to each practice.
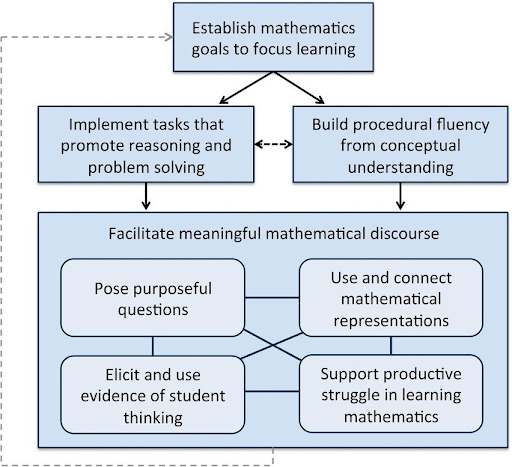
The 8 practices, shown here, work together to ensure students experience high quality math instruction. The structure of this diagram reflects the order in which a teacher engages with the practices. First, they establish goals. Then teachers implement tasks that promote reasoning, problem solving, procedural fluency, and conceptual understanding. Finally, during the lessons, teachers facilitate discourse using the remaining four practices. We have written previously about discourse, so this post focuses on the equitable teaching criteria aligned to the top three effective teaching practices: 1, 2, and 6.
Teaching Practice 1: Establish mathematics goals to focus learning.
From Catalyzing Change, p. 32
Equitable Teaching:
- Establish learning progressions that build students’ mathematical understanding, increase their confidence, and support their mathematical identities as doers of mathematics.
- Establish high expectations to ensure that each and every student has the opportunity to meet the mathematical goals.
- Establish classroom norms that position each and every student as a competent mathematics thinker.
- Establish classroom environments that promote learning mathematics as just, equitable, and inclusive.
A cycle of invitation, deep study, consolidation, and application recurs throughout the curriculum. The invitation begins with the first lesson of every unit, and continues in the structure of every lesson (warm up, activities, cool-down) and activity (launch, activity, synthesis). The sequence intentionally provides access to all learners, while continuing to hold students to the high expectation that they will meet the mathematical goals of grade-level content. It is designed to connect students’ learning to previous lessons, units, and grade levels. The models and representations are chosen carefully and coherently, so students can build their understanding on a solid foundation, rather than numerous disparate ideas.
During an IM lesson, teachers don’t just tell students about the math. The structure of the lessons ensures that all students spend time in deep study. Students spend most of their time in class doing mathematics. A curriculum doesn’t hold the power to make students identify as mathematicians, but the structures can support their mathematical identities as doers of mathematics.
While much of the responsibility for building norms and environments rests on the shoulders of classroom teachers, a curriculum can include aspects that support these ideas. Routines such as Which One Doesn’t Belong, and Notice and Wonder are open, meaning there is no one right answer to the prompt. The teacher is encouraged to value contributions from a wide selection of students through the teacher’s guide, in both the text describing the routine and the sample responses included for each prompt. By including more voices in the discussion, and positioning each perspective as worthy, a teacher can build an environment where more students feel encouraged to learn mathematics.
An example from IM 9-12 Math: Geometry, Unit 1, Lesson 4
| Ask students to share the things they noticed and wondered. Record and display their responses for all to see. If possible, record the relevant reasoning on or near the image. After all responses have been recorded without commentary or editing, ask students, “Is there anything on this list that you are wondering about now?”. Encourage students to respectfully disagree, ask for clarification, or point out contradicting information. |
Things students may notice: • Some intersection points are marked and others aren’t. • There are 7 overlapping circles. • There is a figure that looks like a 6-petaled flower in the center circle. Things students may wonder: • Are all the circles the same size? • How many kinds of shapes are there in the image? • If we connected other intersection points together, what kinds of shapes could we make? |
| The description of the routine specifies that responses should be recorded without commentary or editing. | In the sample responses, noticing a flower is equally as valid as noticing intersection points. |
Teaching Practice 2: Implement tasks that promote reasoning and problem solving.
From Catalyzing Change, p. 32
Equitable Teaching:
- Engage students in tasks that provide multiple pathways for success and that require reasoning, problem solving, and modeling, thus enhancing each student’s mathematical identity and sense of agency.
- Engage students in tasks that are culturally relevant.
- Engage students in tasks that allow them to draw on their funds of knowledge (that is, the resources that students bring to the classroom, including their home, cultural, and language experiences).
Task choice is the thing that curriculum authors have the most power over. Tasks in the curriculum frequently provide multiple pathways and always require reasoning, problem solving, or modeling. In geometry, students can choose to reason through a problem using compass and straightedge, tracing paper, or technology. Throughout the curriculum students are encouraged to choose what approach makes sense to them, so long as they can justify their response.
In IM 6–8 Math, each unit ends with an application problem where modeling techniques frequently come into play. In IM 9–12 Math, there are modeling prompts which provide each student with the freedom to solve the problem their own way and require students to make mathematical assumptions as they reason through the complexity of a loosely defined problem.
Teaching Practice 6: Build procedural fluency from conceptual understanding.
From Catalyzing Change, p. 34
Equitable Teaching:
- Connect conceptual understanding with procedural fluency to help students make meaning of the mathematics and develop a positive disposition toward mathematics.
- Connect conceptual understanding with procedural fluency to reduce mathematical anxiety and position students as mathematical knowers and doers.
- Connect conceptual understanding with procedural fluency to provide students with a wider range of options for entering a task and building mathematical meaning.
One of the benefits of the cycle of invitation, deep study, consolidate, and apply is the way students are continually building on conceptual understanding. The invitation is a chance for students to explore a concept. Teachers connect back to that foundation throughout the rest of the instructional cycle: deep study, consolidate, and apply. Students are asked to show or explain their reasoning throughout the lessons, which helps them make meaning of the mathematics they’re studying. The multiple opportunities stance of this curriculum (as opposed to high stakes, single chance) supports the development of a positive disposition while reducing anxiety.
The materials provided to teachers support them not only in reducing mathematical anxiety, but also in reducing language anxiety and other barriers for students with disabilities. Each lesson includes research-based supports for ELLs from SCALE. These supports provide additional options for entering a task or building mathematical meaning beyond those already embedded in the curriculum for all learners.

So, what impact does curriculum have? Curriculum is not the sole determinant of a student’s mathematical experience, but it is an important factor. IM authors have made numerous intentional choices to promote equitable teaching, and embedded this work in IM K–12 Math. It’s up to schools to provide teachers with the structures and support to implement the curriculum as intended. This year more than ever, all stakeholders need to make a commitment to support equity in teaching. The invitation, additional supports, and student choice remain essential aspects of mathematics learning during a pandemic. Check out resources like the curriculum adaptation packs, available on IM’s Distance Learning portal — which you can learn more about in a blog post by David Petersen and Kate Nowak — to see how IM recommends adapting IM K–12 Math to equitably meet the needs of students, whatever the teaching environment may be.
Next Steps
Join the conversation! Comment below, or join the new IM Community Hub.
Get more strategies and support for distance learning from IM, including the adaptation packs for IM 6–8 Math and IM 9–12 Math referenced in the blog post.
References
National Council of Teachers of Mathematics. (2018). Catalyzing change in high school mathematics: Initiating critical conversations. Reston, VA: NCTM.